|
A. 1 |
|---|
| B. 2 |
| C. 3 |
| D. 4 |
E. 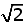 |
[ 6-8726 - op net sinds 5.4.2021-(E)-9.12.2023 ]
Translation in E N G L I S H
Oplossing - Solution
Stel I gelijk aan de gegeven integraal \( \int_{1}^{3}{\frac{\sqrt x}{\sqrt{4-x}+\sqrt x}\;dx}\)
en pas de substitutie 4 − x = t toe
( ⇒ dx = −dt, x = 1 k.o.m. t = 3, x = 3 k.o.m. t = 1)
\(I = \int_{3}^{1}{\frac{\sqrt{4-t}}{\sqrt t+\sqrt{4-t}}(- d\ t)}=\int_{1}^{3}{\frac{\sqrt{4-t}}{\sqrt t+\sqrt{4-t}}\:dt \overset{!!}{=}}\int_{1}^{3}{\frac{\sqrt{4-x}}{\sqrt x+\sqrt{4-x}}\:dx}\)
\(2I = \int_{1}^{3}{\frac{\sqrt x}{\sqrt{4-x}+\sqrt x} d\ x}+\int_{1}^{3}{\frac{\sqrt{4-x}}{\sqrt x+\sqrt{4-x}}\:dx}=\int_{1}^{3}{\frac{\sqrt x+\sqrt{4-x}}{\sqrt{4-x}+\sqrt x}\:dx}\\=\int_{1}^{3}{\:dx}=\left[x\right]_1^3=3-1=2\)
Bijgevolg is de gevraagde integraal \(I\) gelijk aan 1.