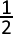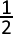![https://latex.codecogs.com/png.image?\dpi{110}\displaystyle \lim_{x \to +\infty} \left [4^n.\left ( 1-\cos \frac{1}{2^n} \right ) \right ]=](https://latex.codecogs.com/png.image?\dpi{155}\fn_jvn&space;\displaystyle&space;&space;\lim_{n&space;\to&space;+\infty}&space;\left&space;[4^n.\left&space;(&space;1-\cos&space;\frac{1}{2^n}&space;\right&space;)&space;\right&space;]=)
|
A. 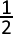 |
|---|
| B. 1 |
| C. 2 |
| D. 4 |
| E. 8 |
[ 6-8721 - op net sinds 4.4.2021-(E)-7.11.2024 ]
Translation in E N G L I S H
![https://latex.codecogs.com/png.image?\dpi{110}\displaystyle \lim_{x \to \infty} \left [4^n.\left ( 1-\cos \frac{1}{2^n} \right ) \right ]=](https://latex.codecogs.com/png.image?\dpi{110}\fn_jvn&space;\displaystyle&space;&space;\lim_{n&space;\to&space;\infty}&space;\left&space;[4^n.\left&space;(&space;1-\cos&space;\frac{1}{2^n}&space;\right&space;)&space;\right&space;]=)
|
A. 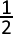 |
|---|
| B. 1 |
| C. 2 |
| D. 4 |
| E. 8 |
Oplossing - Solution
\({\color{Yellow} .} \displaystyle\lim_{n \to +\infty }\; \left [4^n\cdot \left ( 1-cos\, \frac{1}{2^n} \right ) \right ] \quad \begin{matrix} {\color{Yellow} .} \\ _{(1-\cos 2\alpha =2\sin^2\alpha )} \end{matrix} \\ =\displaystyle\lim_{n \to +\infty }\;\left [ 4^n\cdot 2\cdot \sin ^2\frac{1}{2^{n+1}} \right ] \\ =2\cdot \displaystyle\lim_{n \to +\infty }\;\left [ 4^n\left ( \frac{1}{2^{n+1}}\cdot \frac{\sin \frac{1}{2^{n+1}}}{\frac{1}{2^{n+1}}} \right ) ^2\right ] \\e =2\cdot
\displaystyle\lim_{n \to +\infty }\;\left [ 4^n\cdot \frac{1}{2^{2n+2}}\cdot 1^2 \right ] \\ =2\cdot
\displaystyle\lim_{n \to +\infty }\;\frac{4^n}{4^n\cdot 4}=\frac{1}{2}\)