Hoeveel asymptoten
bezit de functie
=\frac{x+7}{|x|-5}\;&space;?)
|
A. 1 |
|---|
| B. 2 |
| C. 3 |
| D. 4 |
| E. geen enkel |
[ 5-7706 - op net sinds 29.8.2020-(E)-1.12.2024 ]
Translation in E N G L I S H
What is the number
of asymptotes
of the function
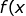=\frac{x+7}{|x|-5}\;&space;?)
|
A. 1 |
|---|
| B. 2 |
| C. 3 |
| D. 4 |
| E. none |
Oplossing - Solution
H.A. : y = −1 en y = 1 [ bv. y = −1 want \(\small\displaystyle \lim_{x \to -\infty}\;\frac{x+7}{|x|-5}=
\displaystyle \lim_{x \to -\infty}\;\frac{x+7}{-x-5}=
\displaystyle \lim_{x \to -\infty}\;\frac{x}{-x}=-1 \) ]
Daar er twee horizontale asymptoten zijn, zijn er geen schuine asymptoten.
V.A. : x = − 5 en x = 5 [de nulwaarden van |x| − 5 zijn immers − 5 en 5]
In totaal zijn er dus vier asymptoten.

=\frac{x+7}{|x|-5}\;&space;?)
