Wiskunde Multiple Choice Vraag uit Gricha's Wiskundige Vragenbank

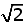
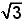
In een cirkel is een regelmatige twaalfhoek ingeschreven die dezelfde oppervlakte heeft als een vierkant. Wat is de verhouding van de zijde van het vierkant tot de straal van de cirkel ?	A. 1
	B. 2
	C. 3
	D.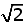
	E.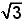

[ 4-6622 - op net sinds 25.3.16-(E)-25.9.2024 ]

Translation in E N G L I S H

In a circle is a regular dodecagon inscribed with the same surface area as a square. What is the ratio of the side of the square to the radius of the circle ?	A. 1
	B. 2
	C. 3
	D.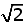
	E.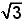

Oplossing - Solution

Weze r de straal van de cirkel en z de zijde van het vierkant.
De oppervlakte van de twaalfhoek is te beschouwen als de som van de oppervlaktes van twaalf gelijkbenige driehoeken met tophoek 30° (360°/12) en opstaande zijden r.
Vandaar dat de totale oppervlakte is : 12.½.r.r.sin30° = 3r².
Zodoende moet 3r² = z² zodat de verhouding ^z/_r gelijk is aan