die 1 −
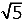 en 1 +
en 1 + 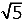
als oplossingen heeft is bv.
|
Een vierkansvergelijking die 1 − 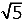 en 1 + en 1 + 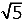 als oplossingen heeft is bv. |
A. x2 − 4x + 2 = 0 |
|---|---|
| B. x2 − 2x − 4 = 0 | |
| C. x2 − 2x + 4 = 0 | |
| D. x2 − 6x + 6 | |
| E. x2 − x − 1 = 0 |
[ 4-4620 - op net sinds 2.8.10-(E)-27.10.2023 ]
|
Which equation has the solutions x = 1 − 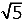 and and x = 1 + 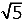 ? ?
|
A. x2 − 4x + 2 = 0 |
|---|---|
| B. x2 − 2x − 4 = 0 | |
| C. x2 − 2x + 4 = 0 | |
| D. x2 − 6x + 6 | |
| E. x2 − x − 1 = 0 |
