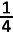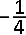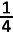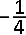Vind je een fout in de opgave, zelfs een spellingsfout of een layout die beter kan of een figuur die niet doorkomt, of een verkeerde vraag bij een opgave : gelieve mij een lege mail te sturen ( gricha@gricha.be ) met alleen in het onderwerp bv. vraag 9876 nakijken a.u.b. Bedankt !

De normaal in het punt ( 1, −2 ) van de parabool y2 = 4x   heeft  als richtingscoëfficiënt A.   1
B. 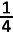 1op4
C. 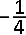 1/4
D.   4
E.  − 4
A    B    C    D    E

[ 6-4079 - op net sinds 31.3.2020-(E)-20.10.2023 ]

Translation in   E N G L I S H

A.  
B.  
C.  
D.  
E.  

Oplossing - Solution

2.y.dy = 4.dx ⇒ afgeleide is dy/dx = 2/y
In het punt ( 1, −2 ) is die afgeleide 2/(−2) = −1
De richtingscoëfficiënt van de raaklijn in dat punt is dus −1
De richtingscoëfficiënt van de normaal in dat punt is bijgevolg 1
(het tegengestelde van het omgekeerde van −1)
gricha