In een rechthoekige driehoek met hoeken A, B en C is de maximale waarde van
cos A + cos B + cos C
cos A + cos B + cos C
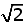
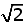
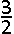
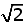
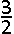
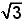
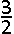
|
In een rechthoekige driehoek met hoeken A, B en C is de maximale waarde van
cos A + cos B + cos C |
A. 1 + 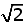 |
|---|---|
B. 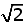 | |
C. 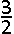 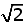 | |
D. 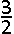 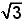 | |
E. 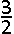 |
[ 5-3984 - op net sinds 17.2.2020-(E)-4.11.2023 ]
|
A, B and C are the measures of the three angles of a right-angled/rectangular triangle. What is the maximum value of cos A + cos B + cos C ? |
A. |
|---|---|
| B. | |
| C. | |
| D. | |
| E. |
