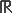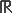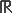De oplossingenverzameling van
− x3 + 6x2 − 13x + 10 < 0
is
|
A. ]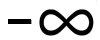 , 2 [ , 2 [ |
|---|
B. ] 2, 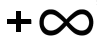 [ [ |
C. ]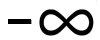 , − 5 [ ∪ ] 1, 2 [ , − 5 [ ∪ ] 1, 2 [ |
D. 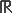 |
E. ] − 5, −1[ ∪ ] 2, 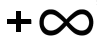 [ [ |
[ 5-2235 - op net sinds 7.10.15-(E)-17.1.2025 ]
Translation in E N G L I S H
The solution set of
−x3 + 6x2 − 13x + 10 < 0
is
|
A. ( 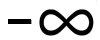 , 2 ) , 2 ) |
|---|
| B. ( 2, ∞ ) |
C. ( 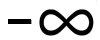 , − 5 ) ∪ ( 1, 2 ) , − 5 ) ∪ ( 1, 2 ) |
D. 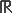 |
| E. ( − 5, −1) ∪ ( 2, ∞ ) |
Oplossing - Solution
V(x) = −x³ + 6x² − 13x + 10
V(2) = −8 + 24 − 26 + 10 = 0
V(x) is dus deelbaar door x − 2.
Het quotiënt vinden we met de regel van HORNER zodat
V(x) = (x − 2).(−x² + 4x − 5)
Daar de discriminant van de tweede factor D = 16 − 20
negatief is, is (−x² + 4x − 5) steeds negatief en is
(x − 2).(−x² + 4x − 5) < 0 ⇔ (x − 2) > 0 ⇔ x > 2