(x − 2)( − x2 + 4x − 5 ) < 0 is
|
De oplossingenverzameling van (x − 2)( − x2 + 4x − 5 ) < 0 is |
A. |
|---|---|
| B. ] 2, | |
| C. ] | |
| D. leeg | |
| E. ] − 1, 2 [ ∪ ] 5 , |
[ 5-0571 - op net sinds 17.10.04-(E)-21.7.2024]
|
The set of solutions of (x − 2)( − x2 + 4x − 5 ) < 0 is |
A. 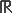 \ { 2 } (or: x ≠ 2 ) \ { 2 } (or: x ≠ 2 ) |
|---|---|
| B. ( 2 , ∞) (or: x > 2 ) | |
| C. ( | |
| D. empty | |
| E. ( − 1 , 2 ) ∪ ( 5, ∞) |
